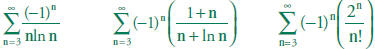The California Mathematics Content Standards
Advanced
Placement
Probability
and Statistics Mathematics Content Standards
This discipline is a technical and in-depth extension of
probability and statistics. In
particular, mastery of academic content for advanced placement gives students
the
background to succeed in the Advanced Placement examination in the
subject.
|
Note: The sample problems illustrate the standards and are written to help clarify them. Some problems are written in a form that can be used directly with students; others will need to be modified before they are used with students. |
1.0 Students solve probability problems with finite
sample spaces by using the rules for addition, multiplication, and complementation for probability distributions and understand the simplifications that arise with independent events. |
|
|
2.0 Students know the definition of conditional probability and use it to solve for probabilities in finite sample spaces. You have 5 coins in your pocket: 1 penny, 2 nickels, 1 dime, and 1 quarter. If you pull out 2 coins at random and they are collectively worth more than 10 cents, what is the probability that you pulled out a quarter? |
||
|
3.0 Students demonstrate an understanding of the notion of discrete random variables by using this concept to solve for the probabilities of outcomes, such as the probability of the occurrence of five or fewer heads in 14 coin tosses. |
||
|
4.0 Students understand the notion of a continuous random variable and can interpret the probability of an outcome as the area of a region under the graph of the probability density function associated with the random variable. Consider a continuous random variable X whose possible values are numbers between 0 and 2 and whose probability density function is given by 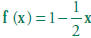 for 0 ≤ x ≤ 2 . What is the
probability that X > 1? for 0 ≤ x ≤ 2 . What is the
probability that X > 1? |
||
|
5.0 Students know the definition of the mean of a discrete random variable and can determine the mean for a particular discrete random variable. |
||
|
6.0 Students know the definition of the variance of a discrete random variable and can determine the variance for a particular discrete random variable. |
||
|
7.0 Students demonstrate an understanding of the standard distributions (normal, binomial, and exponential) and can use the distributions to solve for events in problems in which the distribution belongs to those families. Suppose that X is a normally distributed random variable with mean m = 0. If P(X < c) = 2/3, find P(-c < X < c). |
||
|
8.0 Students determine the mean and the standard deviation of a normally distributed random variable. |
||
|
9.0 Students know the central limit theorem and can use it to obtain approximations for probabilities in problems of finite sample spaces in which the probabilities are distributed binomially. |
||
|
10.0 Students know the definitions of the mean, median, and mode of distribution of data and can compute each of them in particular situations. |
||
|
11.0 Students compute the variance and the standard deviation of a distribution of data. |
||
|
12.0 Students find the line of best fit to a given distribution of data by using least squares regression. |
||
|
13.0 Students know what the correlation coefficient of two variables means and are familiar with the coefficient’s properties. |
||
|
14.0 Students organize and describe distributions of data by using a number of different methods, including frequency tables, histograms, standard line graphs and bar graphs, stem-and-leaf displays, scatterplots, and box-and-whisker plots. |
||
|
15.0 Students are familiar with the notions of a statistic of a distribution of values, of the sampling distribution of a statistic, and of the variability of a statistic. |
||
|
16.0 Students know basic facts concerning the relation between the mean and the standard deviation of a sampling distribution and the mean and the standard deviation of the population distribution. |
||
|
17.0 Students determine confidence intervals for a simple random sample from a normal distribution of data and determine the sample size required for a desired margin of error. |
||
|
18.0 Students determine the P-value for a statistic for a simple random sample from a normal distribution. |
||
|
19.0 Students are familiar with the chi-square distribution and chi-square test and understand their uses. |
Calculus Mathematics Content Standards
When taught in high school, calculus should be presented
with the same level of depth and
rigor as are entry-level college and university calculus courses. These
standards outline a
complete college curriculum in one variable calculus. Many high school programs
may
have insufficient time to cover all of the following content in a typical
academic year. For
example, some districts may treat differential equations lightly and spend
substantial time
on infinite sequences and series. Others may do the opposite. Consideration of
the College
Board syllabi for the Calculus AB and Calculus BC sections of the Advanced
Placement
Examinations in Mathematics may be helpful in making curricular decisions.
Calculus is a
widely applied area of mathematics and involves a beautiful intrinsic theory.
Students
mastering this content will be exposed to both aspects of the subject.
|
1.0 Students demonstrate knowledge of both the formal definition and the graphical interpretation of limit of values of functions. This knowledge includes one-sided limits, infinite limits, and limits at infinity. Students know the definition of convergence and divergence of a function as the domain variable approaches either a number or infinity: 1.1 Students prove and use theorems evaluating the limits of sums, products, quotients, and composition of functions. 1.2 Students use graphical calculators to verify and estimate limits. 1.3 Students prove and use special limits, such as the limits of (sin(x))/x and (1-cos(x))/x as x tends to 0. Evaluate the following limits, justifying each step:
|
Note: The sample problems illustrate the standards and are written to help clarify them. Some problems are written in a form that can be used directly with students; others will need to be modified before they are used with students. |
|
|
2.0 Students demonstrate knowledge of both the formal definition and the graphical interpretation of continuity of a function. For what values of x is the function 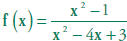 continuous? Explain. continuous? Explain. |
||
|
3.0 Students demonstrate an understanding and the application of the intermediate value theorem and the extreme value theorem. |
||
|
4.0 Students demonstrate an understanding of the formal definition of the derivative of a function at a point and the notion of differentiability: 4.1 Students demonstrate an understanding of the derivative of a function as the slope of the tangent line to the graph of the function. 4.2 Students demonstrate an understanding of the interpretation of the derivative as an instantaneous rate of change. Students can use derivatives to solve a variety of problems from physics, chemistry, economics, and so forth that involve the rate of change of a function. 4.3 Students understand the relation between differentiability and continuity. 4.4 Students derive derivative formulas and use them to find the derivatives of algebraic, trigonometric, inverse trigonometric, exponential, and logarithmic functions. Find all points on the graph of 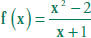 where the tangent line is parallel
where the tangent line is parallelto the tangent line at x = 1. |
||
|
5.0 Students know the chain rule and its proof and applications to the calculation of the derivative of a variety of composite functions. |
||
|
6.0 Students find the derivatives of parametrically defined functions and use implicit differentiation in a wide variety of problems in physics, chemistry, economics, and so forth. For the curve given by the equation 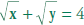 , use implicit differentiation , use implicit differentiationto find 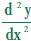 . . |
||
|
7.0 Students compute derivatives of higher orders. |
||
|
8.0 Students know and can apply Rolle’s theorem, the mean value theorem, and L’Hôpital’s rule. |
||
|
9.0 Students use differentiation to sketch, by hand, graphs of functions. They can identify maxima, minima, inflection points, and intervals in which the function is increasing and decreasing. |
||
|
10.0 Students know Newton’s method for approximating the zeros of a function. |
||
|
11.0 Students use differentiation to solve optimization (maximum-minimum problems) in a variety of pure and applied contexts. A man in a boat is 24 miles from a straight shore and wishes to reach a point 20 miles down shore. He can travel 5 miles per hour in the boat and 13 miles per hour on land. Find the minimal time for him to reach his destination and where along the shore he should land the boat to arrive as fast as possible. |
||
|
12.0 Students use differentiation to solve related rate problems in a variety of pure and applied contexts. |
||
|
13.0 Students know the definition of the definite integral by using Riemann sums. They use this definition to approximate integrals. The following is a Riemann sum that approximates the area under the graph of a function f(x), between x = a and x = b. Determine a possible formula for the function f(x) and for the values of a and b:
|
||
|
14.0 Students apply the definition of the integral to model problems in physics, economics, and so forth, obtaining results in terms of integrals. |
||
|
15.0 Students demonstrate knowledge and proof of the fundamental theorem of calculus and use it to interpret integrals as antiderivatives.
If |
||
|
16.0 Students use definite integrals in problems involving area, velocity, acceleration, volume of a solid, area of a surface of revolution, length of a curve, and work. |
||
|
17.0 Students compute, by hand, the integrals of a wide variety of functions by using techniques of integration, such as substitution, integration by parts, and trigonometric substitution. They can also combine these techniques when appropriate. Evaluate the following: 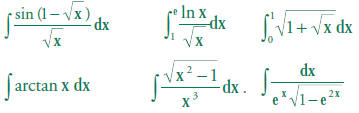 |
||
|
18.0 Students know the definitions and properties of inverse trigonometric functions and the expression of these functions as indefinite integrals. |
||
|
19.0 Students compute, by hand, the integrals of rational functions by combining the techniques in standard 17.0 with the algebraic techniques of partial fractions and completing the square. |
||
|
20.0 Students compute the integrals of trigonometric
functions by using the techniques noted above. |
||
|
21.0 Students understand the algorithms involved in Simpson’s rule and Newton’s method. They use calculators or computers or both to approximate integrals numerically. |
||
|
22.0 Students understand improper integrals as limits of definite integrals. |
||
|
23.0 Students demonstrate an understanding of the definitions of convergence and divergence of sequences and series of real numbers. By using such tests as the comparison test, ratio test, and alternate series test, they can determine whether a series converges. Determine whether the following alternating series converge absolutely, converge conditionally, or diverge:
|
||
|
24.0 Students understand and can compute the radius (interval) of the convergence of power series. |
||
|
25.0 Students differentiate and integrate the terms of a power series in order to form new series from known ones. |
||
|
26.0 Students calculate Taylor polynomials and Taylor series of basic functions, including the remainder term. |
||
|
27.0 Students know the techniques of solution of selected elementary differential equations and their applications to a wide variety of situations, including growth-and-decay problems. |





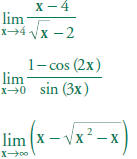
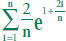 .
.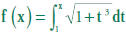 find f'(2).
find f'(2).