Quadratic Equations, Inequalities and Functions
Chapter 11: Quadratic Equations, Inequalities and
Functions
• Discuss different methods of solving quadratic equations
• Methods for graphing quadratic equations
• Applications of quadratic equations
Section 11.1: Solving Quadratic Equations using the
Square Root Property
• Quadratic equations come in the form of ax2 + bx + c = 0 ;
standard form
• To be able to solve a quadratic equation, it must be in standard form
Zero Factor Property
• If two numbers have a product of zero, then at least one of the numbers
must be zero.
• We use this property to solve equations after they have been factored
• Once the equation is factored we set each factor equal to zero and then solve
the
individual equations.
Example: Use the zero factor property to solve the
given quadratic equation.
2x2 − 3x = −1
Square Root Property
• If k is a positive number and if x2 = k , then
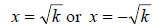
The solution can also be written as 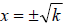
• If the quadratic equation is of the form x2 = k then the square
root property can be used
Examples: Solve each equation. Write radicals in
simplified form.
a) z 2 = 49
b) x2 = 12
c) 3x2 − 8 = 88
d) (p − 4)2 = 3
e) (5m +1)2 = 7
f) x2 = −17
g) (x + 5)2 = −100
Section 11.2: Solving Quadratic Equations by Completing
the Square
• A method for solving quadratic equations
• The goal is to factor the left side of the quadratic equation so that it is a
perfect square
• The right side of the equation is a constant
• The square root property is the used to finish the solving of the equation
• We start with an equation in standard form ax2 + bx + c = 0
• The end result is of the form (x + k )2 = n
Steps to Complete the Square
1. Make sure a is 1. If a is not 1, then perform the proper division
2. Write in the form ax2 + bx = −c ; variable terms on the left,
constant term on the right
3. Complete the square using the formula 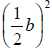
4. Add the value found in step 3 to both sides of the equation
5. Factor the left side as a perfect square, simplify the right side
6. Use the square root property to solve
Examples: Solve the given equations using
completing the square.
a) x2 − 2x −10 = 0
b) x2 + 4x = 1
c) 4k2 − 24k +11 = 0
d) 5t 2 −15t +12 = 0
Section 11.3: Solving Quadratic Equations by the Quadratic Formula
• Another method for solving quadratic equations
• Before the quadratic formula can be used the quadratic equation must be in
standard form
Quadratic Formula
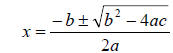
Examples: Use the quadratic formula to solve the
given quadratic equations.
a) 4x2 −11x − 3 = 0
b) 2x2 +19 = 14x
c) (x + 5)(x +1) = 10x
Discriminant
• A part of the quadratic formula; b2 − 4ac
• The discriminant can be used to determine the number and type of solutions a
quadratic
equation has
• The following table lays out the types of solutions
| Discriminant | Number and Type of Solutions |
| Positive, and the square of an integer | 2 rational solutions |
| Positive, but not the square of an integer | 2 irrational solutions |
| Zero | 1 rational solution |
| Negative | 2 non-real complex solutions |
Examples: Find each discriminant. Use it to predict
the number and type of solutions for each
equation.
a) 10x2 − x − 2 = 0
b) 3x2 − x = 7
c) 16x2 + 25 = 40x
Example: Find k so that the equation will have
exactly one rational solution.
x2 − kx + 64 = 0
Section 11.4: Equations in Quadratic Form
• We can solve quadratic equations by 4 different methods
-Factoring
-Square Root Property
-Completing the Square
-Quadratic Formula
• Some equations can be simplified down to quadratic form
Example: Solve the given equations.
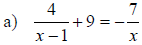
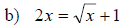
Example: Solve the given application problem using
the six step method discussed previously.
1. In 1 ¾ hours Sammy rows his boat 5 miles up the river and comes back. The
speed of the
current is 3 mph. How fast does Sammy row?
Section 11.5: Formulas and Further Applications
• This section illustrates the many uses of quadratic equations
• We also look at formulas
Examples: Solve the given formulas for the
specified variable.
a) A =πr 2 for r
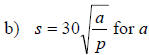
c) 2t2 − 5t + k = 0 for t
• Recall the Pythagorean Relation, c2 = a2 + b2
Example: A ladder is leaning against a house. The
distance from the bottom of the ladder to the
house is 5 ft. The distance from the top of the ladder to the ground is 1 ft
less than the length of
the ladder. How long is the ladder?
Example: A ball is projected upward from the
ground. Its distance in feet from the ground at t
seconds is s(t) = −16t2 + 64t .
a) At what time will the ball hit the ground?
b) At what time will the ball be 32 ft from the ground?
Section 11.6: Graphs of Quadratic Functions
• We now look at how to graph quadratic equations
• In this section we look at horizontal shifts and vertical shifts associated
with parabolas
• We can also use a table of values to plot points and determine the graph of
quadratic
equations
• A quadratic function is of the form f (x) = ax2 + bx + c ; standard
form
• A quadratic function can also be in the form of f (x) = a(x − h)2 +
k
• Functions of the form f (x) = x2 + k have a vertical shift of k
units up if k is positive and
k units down if k is negative
• Functions of the form f (x) = (x − h)2 have a horizontal shift of h
units to the right if h is
positive and h units to the left if h is negative
• Recall the ideas of domain and range; interval notation
• Recall the general shape of quadratic functions; f (x) = x2
General Principles
1. Graph the quadratic function defined by f (x) = a(x − h)2 + k
is a parabola with vertex
(h, k)
2. The graph opens up if a is positive and down if a is negative
3. The graph is wider than that of f (x) = x2 if 0 <| a |< 1. The
graph is narrower than that of
f (x) = x2 if | a |> 1
Examples: Graph the following functions. In each case state the
horizontal shift, the vertical
shift, the vertex, how the graph opens and its width in terms of f (x) = x2
.
a) f (x) = x2 + 3
b) f (x) = −x2 +1
c) f (x) = x2 −1
d) f (x) = (x + 2)2
e) f (x) = (x − 3)2
f) f (x) = −(x −1)2
g) f (x) = (x − 2)2 +1
h) f (x) = −3(x +1)2 − 2
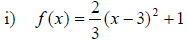
Section 11.7: More about Parabolas and Their Applications
• We use a similar set of principles when graphing quadratic equations in
standard form
• To determine the vertex of the parabola, we use the following formula

General Principles of a Quadratic Function in Standard Form
1. Determine whether the graph opens up or down. If a > 0 the parabola opens
up. If
a < 0the parabola opens down.
2. Find the vertex using the vertex formula.
3. Find the x and y intercepts. Recall that an x intercept is found when y is
replaced with
zero and the equation is solved for x. A y intercept is found when x is replaced
with zero
and the equation is solved for y; this usually involves factoring.
4. Graph the vertex and intercepts. Plot additional points as needed.
Example: Graph the given quadratic equation. Use
the general principles as described above.
Also, determine the domain and range.
f (x) = x2 − 6x + 5
• We can use the vertex formula to solve applications of quadratic equations
• Application problems which involve maximum or minimum areas require the use of
the
vertex formula
• Recall that the vertex of the parabola can be thought of as its maximum point
or its
minimum point which enables us to use the vertex formula when determining a
maximum or minimum value of a quadratic equation
Example: Use the vertex formula to solve the
following application problems.
1. A farmer has 100 ft of fencing. He wants to put a fence around the
rectangular field next
to a building. Find the maximum area he can enclose and the dimensions of the
field
when the area is maximized.
2. A toy rocket is launched from the ground so that its distance in feet above
the ground
after t seconds is s(t) = −16t 2 + 208t . Find the maximum height it
reaches and the
number of seconds it takes to reach that height.




